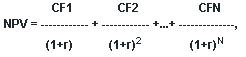Калькулятор для расчета внутренней нормы доходности

Внутренняя норма доходности (IRR) Калькулятор

Внутренняя норма доходности (IRR) Калькулятор

Внутренняя норма доходности является одним из наиболее часто используемых мер для оценки инвестиций.Инвестиции с более высокой внутренней норме доходности считается более выгодным, чем инвестиции с низкой внутренней нормы доходности. Этот бесплатный онлайн инструмент поможет вам рассчитать IRR, он также генерирует динамический график, чтобы продемонстрировать взаимосвязь между NPV и ставки дисконтирования.

год	Денежный поток в	Денежный поток из (минус)	Поток Чистые денежные средства
<<$index + 1>>
общий:

IRR результат и график

Использование внутренней нормы доходности (IRR) калькулятор

• Внутренняя норма доходности (IRR) — IRR является скорость, чтобы NPV равную нулю в инвестиции
• первоначальных инвестиций — Первоначальные инвестиции на первом году
• Cash-In — Годовой денежный в потоков
• Cash-Out — Годовой денежный вне потоков
• Flow Чистые денежные средства — Прием наличных минус обналичить

Что такое IRR (внутренняя норма доходности)

Как рассчитать IRR?

Это почти невозможно говорить о IRR без упоминания NPV. NPV формула выглядит следующим образом:

Поскольку IRR является скорость, чтобы NPV = 0, мы получаем следующие функции:

или
PV пособия — PV затрат = 0

г является IRR, как только неизвестно, она может быть решена с помощью методов численного или графического анализа.

Давайте посмотрим на пример:
$ 85000 инвестиций возвращается $ 20000 в год в течение 5 лет жизни, что норма прибыли на инвестиции?
Решение:
20000 / (1 + I) + 20000 / (1 + I) ^ 2 + 20000 / (1 + I) ^ 3 + 20000 / (1 + I) ^ 4 + 20000 / (1 + I) ^ 5 = 85000
IRR является 5,68%.

Источник

Internal Rate of Return (IRR) Calculator

How to Calculate Internal Rate of Return

Internal rate of return is a discount rate that is used in project analysis or capital budgeting that makes the net present value (NPV) of future cash flows exactly zero. If you aren’t quite familiar with NPV, you may find it best to read through that article first, as the formula is exactly the same. The difference here is that, instead of summing future cash flows, this time we set the net present value equal to zero, and then we solve for the discount rate. Remember that the discount rate is the rate of return we could expect from alternative projects; therefore, when comparing similar projects, it is generally more desirable to undertake the project with the higher IRR given other common parameters.

• C = Cash Flow at time t
• IRR = discount rate/internal rate of return expressed as a decimal
• t = time period

If we think about things intuitively, if one project (assume all other things equal) has a higher IRR, then it must generate greater cash flows, i.e. a bigger numerator must be divided by a bigger denominator, and hence IRR, given the same initial costs. We can also think of the IRR as the expected compound rate of return of a project. While the cash flows may vary, you only have one IRR per project, because here we are calculating a discount rate that is the same for each year. The difficult part about this calculation is that there is not a straightforward way to solve for IRR using this equation. Your options are either to take a “plug and chug” approach until you hone in on a close approximation, or you may use a calculator. Let’s take a look at an example using the “plug and chug” approach, as using a calculator is straightforward once you understand how to solve for IRR.

Example

Suppose you as the investor are weighing two different potential investments, both of which may positively help your business. You are hoping that, over a three-year period, a new piece of machinery will allow your workers to produce widgets more efficiently, but you are not sure which new machine will be best. One machine costs $500,000 for a three-year lease, and another machine costs $400,000, also for a three-year lease. Let’s call the first machine “Machine 1,” and let’s call the second machine “Machine 2.” Your analysts are projecting increasing cash flows for each machine, as it may take time for your employees to become familiar with the new machine. They are projecting that Machine 1 will produce cash flows of $210,000 in Year 1, $237,000 in Year 2, and $265,000 in Year 3, and they are projecting that Machine 2 will produce cash flows of $181,000 in Year 1, $190,000 in Year 2, and $203,000 in Year 3. You want to calculate the IRR for each project to help determine which machine to purchase.

It is usually easiest both to see and set up the calculation by looking at a table of cash flows. You might consider setting up a table for Machine 1 that looks something like that of the one below:

Year	0	1	2	3
CF	$(500,000)	$210,000	$237,000	$265,000

Remember that at time 0 (the present day) you must outlay $500,000 in order to receive the new piece of machinery, and the following years you will receive cash due to an increase in production of widgets. Now that we have a good visual of what the project looks like financially, let’s set up our equation.

Now we must “solve” this equation for IRR, but we cannot solve this equation like most. We will use the “plug and chug” approach, as mentioned before, in order to get the right side of the equation to match the left, which is zero. Let’s try 20%. So, we plug in 0.20 where IRR is, and we calculate the right side of this equation to be -$7,060.19. In the grand scheme of things, this is close, but we discounted our cash flows too much if we have a negative NPV. This means in our second guess, we must try a slightly lower rate. Since we were relatively close, let’s try 19% this time. Now, we plug in 0.19 where IRR is, and we calculate the right side of the equation to be $1,086.84. We are closer, but we now have underestimated our rate since our NPV is positive. Let’s bump up our rate to 19.2%. This gives an NPV of $-560.62. Again, closer, but we overshot this time. Going back and forth takes some time, but after enough tries, you will get an IRR of roughly 19.13%. To check this, plug in 0.1913 to our original equation, and you will get an NPV quite close to $0. This means that, given our projected cash flows for Machine 1, our discount rate that returns a net present value of approximately $0 is 19.13%.

We will approach Machine 2 similarly. Let’s again start with a table that looks like the one above.

Year	0	1	2	3
CF	$(400,000)	$181,000	$190,000	$203,000

This time, however, we will outlay $400,000 initially. Since we have a good visual of the project financially, we can now set up our equation.

We must solve this equation again by the “plug and chug” approach. Let’s again start with 20% as our rate just to see where we stand. Once we work through our calculations, we get an NPV of $254.63, which in the scheme of things is very close to zero. This means that, while we have underestimated our IRR slightly, we are certainly close. If we nudge that number just slightly to 20.04%, we calculate a net present value of -$0.95, which is certainly close to zero. We can be confident with an IRR of 20.04%, but if you are really picky, you may work even further to get a more exact IRR (in this case, you would get something like 20.03985%). This means that our IRR, or compound rate of return, for Machine 2 is 20.04%. If you as the investor were strictly making your decision based on IRR, you would purchase Machine 2, as this machine would generate higher cash flows relative to its cost.

In general, sometimes we may not get so lucky with our initial guess, and therefore you would have to work through several different iterations of this process in order to hone in on an approximate IRR, but the steps each time will be the same. If you calculate a positive NPV, try increasing your rate, and if you calculate a negative NPV, try decreasing your rate. With time, you will get close. Many times, if you are using this approach, it may be easiest to work in a spreadsheet where you can quickly adjust your rate. By setting up a table like the ones above, you can quickly discount each cash flow and sum them. Once your sum equals zero, you have an accurate IRR for the project.

One other quick thing to note is that, using the IRR formula, you may have more than one IRR. The interesting thing is that, mathematically, both calculations are correct. You will run into multiple roots when your cash flows change sign more than once. Perhaps you may want to read up on Descartes’ rule of signs to better understand the math behind this. In the examples above, you start out with a negative cash flows and then have all positive cash flows, meaning that you will have only one root because the sign changed only once, from a negative cash flow to positive cash flows. If, say, you had a negative cash flow in Year 2, then you would have at most three roots to the equation because you go from a negative cash flow to a positive cash flow, to a negative cash flow, and then back to positive cash flow. Hence, you have three sign changes. If this were the case, it is usually best to graph the polynomial to see at what points your NPV would be positive. You would strive for a cost of capital at the points where NPV is positive.

Источник

Калькулятор для расчета внутренней нормы доходности

Бесплатные статьи и программы для профессионалов и тех кто ценит свое время.

Расчет чистого дисконтированного дохода NPV и внутренней нормы доходности IRR теперь не проблема!

Почему представленные программы на нашем портале заслужили хорошие оценки поисковых систем ? Потому что: все представление программное обеспечение прошло тщательную проверку на совместимость с современными операционными системами и проверку на вирусы. Мы делаем ваш поиск безопасным. Цените бесплатное и качественное.

Понравилась эта статья ? Добавляй её в социальные закладки!
Помни, что именно от тебя зависит что будет на страницах интернета завтра!

Не дай рекламе завоевать интернет, цени бесплатное и качественное .

IRR — Internal Rate of Return показатель представляет собой разницу между всеми денежными притоками и оттоками, приведенными к текущему моменту времени (моменту оценки инвестиционного проекта). Он показывает ту величину денежных средств, которую инвесторы ожидают получить от своего проекта (портфеля), после того, как денежные притоки окупят его первоначальные инвестиционные затраты и последующие денежные оттоки, связанные с реализацией инвестиционного проекта. Так как денежные платежи оцениваются с учетом их временной стоимости и рисков, IRR — Internal Rate of Return можно выразить, как стоимость, добавляемую проектом. Ее также можно охарактеризовать как общую прибыль инвестора.

Формула расчета чистого дисконтированного дохода или чистой приведенной стоимости NPV (Net Present Value). Поступления денежных потоков группируются и суммируются внутри определенных временных периодов. Например, помесячно, поквартально или годично. Тогда, для денежного потока, состоящего из периодов N (шагов), можно представить в виде следующей формулы:

CF = CF1 + CF2 + … + CFN,

То есть, полный денежный поток равен сумме денежных потоков всех периодов. Формула расчета чистого дисконтированного дохода NPV при этом выглядит следующим образом:

Где D — ставка дисконтирования. Она показывает скорость изменения стоимости денег со временем, чем выше ставка дисконтирования, тем выше скорость.

npv формула пример: В случае оценки инвестиций формула расчета записывается в виде:
CF1 CF2 CFN
NPV = -CF0 + ———— + ——— +. + ————-,
(1+r) (1+r) 2 (1+r) N

Где CF0 — инвестиции сделанные на начальном этапе.
Величина CFK/(1+r) N называется дисконтированным денежным потоком на шаге N.
Множитель 1/(1+r) N , используемый в формуле расчета NPV, уменьшается с ростом N, что отражает уменьшение стоимости денег со временем.

Формула расчета NVP может быть использована для оценки уже сделанных в прошлом инвестиций и полученных при этом доходов. В этом случае ставка дисконтирования будет отрицательна, а множитель 1/(1+r) N будет расти с ростом N.

Внутренняя норма доходности, её так же называют внутренней нормой прибыли, либо внутренним коэффициентом окупаемости (IRR — Internal Rate of Return ) — есть норма полученной прибыли, от вложенной инвестиции. Это та норма прибыли (барьерная ставка, ставка дисконтирования), при которой чистая приведенная (текущая) стоимость инвестиции будет нулевой, т.е. равна 0, или это та ставка дисконта, при которой дисконтированные доходы от проекта равны инвестиционным затратам. IRR — Internal Rate of Return выявляет наилучшую ставку дисконта, при котором можно вкладывать капитал без каких-либо потерь и с минимальным риском для собственников, то есть :

IRR = r, при котором NPV = f(r) = 0

Год	Приток платежей	Отток платежей
0		7500
1	2200
2	2500
3	2900

Ставка дисконта r = 10%

NPV = -7500 +2200/(1+10/100) 1 +2500/(1+10/100) 2 +2900/(1+10/100) 3 = -1257.3

-7500 +2200/(1+r/100) 1 +2500/(1+r/100) 2 +2900/(1+r/100) 3 = 0

Чтобы решить данный пример с помощью нашего финансового калькулятора необходимо просто заполнить графы притока и оттока финансовых поступлений (денежной наличности) и через секунду задача решена и Вы знаете какой чистый дисконтированный доход ожидать от инвестиций!

Excel таблица-калькулятор Расчета инвестиций Финансовые функции и таблицы подстановки

Скачать бесплатно без рекламы и ожиданий по прямой ссылке

Источник